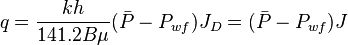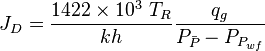Difference between revisions of "JD"

Latest revision as of 14:53, 7 April 2025

Brief

JD - dimensionless productivity index^[1], inverse of dimensionless pressure (based on average pressure) which contains the type of flow regime, boundary condition, drainage shape and stimulation ^[2].

Math & Physics

{J_D} = \frac{1}{\bar{P}_D}

From the Darcy's law for the unfractured well the JD is:

	Well in circular drainage area	Well in a drainage area with the shape factor ${C_A}$ ^[2]
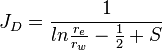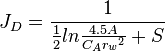Steady state	${J_D} = \frac{1}{ln{\frac{r_e}{r_w}-\frac{1}{2}+S}}$	${J_D} = \frac{1}{\frac{1}{2}ln{\frac{4.5A}{C_A{r_w}^2}+S}}$
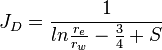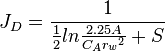Pseudo steady state	${J_D} = \frac{1}{ln{\frac{r_e}{r_w}-\frac{3}{4}+S}}$	${J_D} = \frac{1}{\frac{1}{2}ln{\frac{2.25A}{C_A{r_w}^2}+S}}$

Some typical 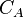${C_A}$ values: circle 31.6, square 30.88 ^[3].

Oil

{J_D} = \frac{141.2 B \mu}{kh} \frac{q}{\bar{P} - P_{wf}} = \frac{141.2 B \mu}{kh} J

{q} = \frac{kh}{141.2 B \mu} (\bar{P} - P_{wf}) J_D = (\bar{P} - P_{wf}) J

Gas

J_D=\frac{1422 \times 10^3\ T_R}{kh} \frac{q_g}{P_{\bar{P}}-P_{P_{wf}}}

Maximum $J_D$

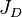
The undamaged unstimulated vertical well potential in a pseudo steady radial flow in a circular drainage area:

{J_D}_{max} \approx \frac{1}{ln{\frac{500}{0.1}-\frac{3}{4}+0}} \approx 0.1287

The maximum possible stimulated well potential for pseudo steady linear flow is:

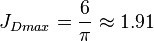${J_D}_{max}= \frac{6}{\pi} \approx 1.91$ , see 6/π stimulated well potential

The maximum possible stimulated well potential for steady state linear flow is:

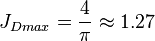${J_D}_{max}= \frac{4}{\pi} \approx 1.27$ , see 4/π stimulated well potential

Nomenclature

B

= formation volume factor, bbl/stb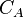

C_A

= Dietz shape factor, dimensionless

J

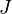= productivity index, stb/psia

J_D

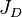= dimensionless productivity index, dimensionless

kh

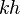= permeability times thickness, md*ft

\bar{P}

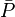= average reservoir pressure, psia

\bar{P}_D

= dimensionless pressure (based on average pressure), dimensionless

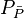P_{\bar{P}}

= average reservoir pseudopressure, psia²/cP

P_{wf}

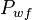= well flowing pressure, psia

P_{P_{wf}}

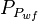= average well flowing pseudopressure, psia²/cP

q

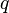= flowing rate, stb/d

q_g

= gas rate, MMscfd

r_w

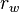= wellbore radius, ft

r_e

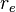= drainage radius, ft

S

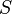= skin factor, dimensionless

T

= temperature, °R

Greek symbols

\mu

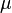= viscosity, cp

References

↑ Rueda, J.I.; Mach, J.; Wolcott, D. (2004). "Pushing Fracturing Limits to Maximize Producibility in Turbidite Formations in Russia" (SPE-91760-MS). Society of Petroleum Engineers.
↑ ^2.0 ^2.1 Wolcott, Don (2009). Applied Waterflood Field Development. Houston: Energy Tribune Publishing Inc.
↑ Dietz, D.N. (1965). "Determination of Average Reservoir Pressure From Build-Up Surveys" (SPE-1156-PA). J Pet Technol.

By Mikhail Tuzovskiy on 20250407145307

[pushing-1] Rueda, J.I.; Mach, J.; Wolcott, D. (2004). "Pushing Fracturing Limits to Maximize Producibility in Turbidite Formations in Russia" (SPE-91760-MS). Society of Petroleum Engineers.

[DW-2] 2.0 ^2.1 Wolcott, Don (2009). Applied Waterflood Field Development. Houston: Energy Tribune Publishing Inc.

[Dietz-3] Dietz, D.N. (1965). "Determination of Average Reservoir Pressure From Build-Up Surveys" (SPE-1156-PA). J Pet Technol.

[1]

[2]

[3]

@@ Line 38: / Line 38: @@
 :<math> {J_D} = \frac{141.2 B \mu}{kh} \frac{q}{\bar{P} - P_{wf}} = \frac{141.2 B \mu}{kh} J</math>
-:<math> {q} = \frac{kh}{141.2 B \mu} (\bar{P} - P_{wf}) J_D </math>
+:<math> {q} = \frac{kh}{141.2 B \mu} (\bar{P} - P_{wf}) J_D = (\bar{P} - P_{wf}) J</math>
 ===Gas===
@@ Line 45: / Line 45: @@
 ==Maximum <math>J_D</math>==
-The undamaged unstimulated vertical well potential in a pseudo steady radial flow is:
+The undamaged unstimulated vertical well potential in a pseudo steady radial flow in a circular drainage area:
-:<math> {J_D}_{max} \approx \frac{1}{ln{\frac{500}{0.1}-\frac{3}{4}+0}} \approx 0.13</math>
+:<math> {J_D}_{max} \approx \frac{1}{ln{\frac{500}{0.1}-\frac{3}{4}+0}} \approx 0.1287</math>
 The maximum possible stimulated well potential for pseudo steady linear flow is:
@@ Line 82: / Line 82: @@
 * [[Darcy's law]]
-* [[JD]]
+* [[IPR]]
 * [[Productivity index|J]]
 * [[Production Potential]]
@@ Line 133: / Line 133: @@
 |description=JD dimensionless productivity index
 }}
+<div style='text-align: right;'>By Mikhail Tuzovskiy on {{REVISIONTIMESTAMP}}</div>

Navigation menu

Navigation

Categories

Products