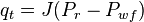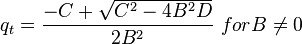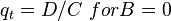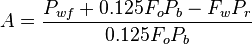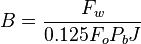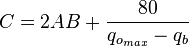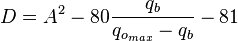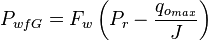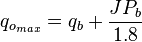Difference between revisions of "3 Phase IPR"

From wiki.pengtools.com
Jump to: navigation, search
(→‎For PwfG wf b)
(→‎Math and Physics)
Line 28: Line 28:
 
:<math> D=A^2-80\frac{q_b}{q_{o_{max}}-q_b}-81</math>
 
:<math> D=A^2-80\frac{q_b}{q_{o_{max}}-q_b}-81</math>
  
−
and:
+
===0 < P<sub>wf</sub> < For P<sub>wfG</sub>===
 +
 
 +
===And===
 
:<math> P_{wfG}=F_w \left ( P_r - \frac{q_{o_{max}}}{J}\right )</math>
 
:<math> P_{wfG}=F_w \left ( P_r - \frac{q_{o_{max}}}{J}\right )</math>
 
:<math> q_{o_{max}}=q_b+\frac{JP_b}{1.8}</math>
 
:<math> q_{o_{max}}=q_b+\frac{JP_b}{1.8}</math>
−
 
−
===0 < P<sub>wf</sub> < For P<sub>wfG</sub>===
 
  
 
==IPR calculator software==
 
==IPR calculator software==

Revision as of 08:36, 11 April 2019

Three-phase Inflow Performance Relationship

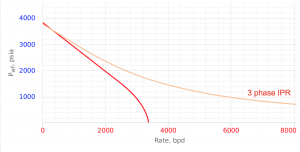
3 Phase IPR Curve [1]

3 Phase IPR calculates IPR curve for oil wells producing water.

3 Phase IPR equation was derived by Petrobras based on combination of Vogel's IPR equation for oil flow and constant productivity for water flow [1].

3 Phase IPR curve is determined geometrically from those equations considering the fractional flow of oil and water [1].

Math and Physics

Total flow rate equations:

For Pb < Pwf < Pr

For pressures between reservoir pressure and bubble point pressure:

q_t =J (P_r - P_{wf})

For PwfG < Pwf < Pb

For pressures between the bubble point pressure and the flowing bottom-hole pressures:

q_t =\frac{-C+\sqrt{C^2-4B^2D}}{2B^2}\ for B \ne 0
q_t =D/C\ for B = 0

where:

A=\frac{P_{wf}+0.125F_oP_b-F_wP_r}{0.125F_oP_b}
B=\frac{F_w}{0.125F_oP_bJ}
C=2AB+\frac{80}{q_{o_{max}}-q_b}
D=A^2-80\frac{q_b}{q_{o_{max}}-q_b}-81

0 < Pwf < For PwfG

And

P_{wfG}=F_w \left ( P_r - \frac{q_{o_{max}}}{J}\right )
q_{o_{max}}=q_b+\frac{JP_b}{1.8}

IPR calculator software

Nomenclature

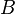B = formation volume factor, bbl/stb
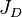J_D = dimensionless productivity index, dimensionless
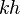kh = permeability times thickness, md*ft
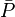\bar{P} = average reservoir pressure, psia
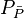P_{\bar{P}} = average reservoir pseudopressure, psia2/cP
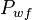P_{wf} = well flowing pressure, psia
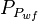P_{P_{wf}} = average well flowing pseudopressure, psia2/cP
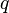q = flowing rate, stb/d
q_g = gas rate, MMscfd
T = temperature, °R

Greek symbols

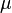\mu = viscosity, cp

References

  1. ↑ 1.0 1.1 1.2 Brown, Kermit (1984). The Technology of Artificial Lift Methods. Volume 4. Production Optimization of Oil and Gas Wells by Nodal System Analysis. Tulsa, Oklahoma: PennWellBookss. 

See also

141.2 derivation
Darcy's law
JD
Production Potential
Retrieved from "https://wiki.pengtools.com/index.php?title=3_Phase_IPR&oldid=6073"