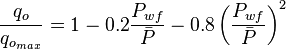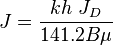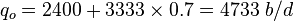Vogel's IPR

Vogel's Inflow Performance Relationship

Vogel's IPR

Vogel's IPR is an empirical two-phase (oil + gas) inflow performance relationship correlation published in 1968 .

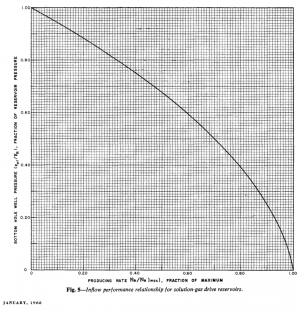
Vogel's IPR is based on computer simulations to several solution gas drive reservoirs for different fluid and reservoir relative permeability properties.

Vogel's IPR is the default IPR correlation to calculate oil wells performance in the PQplot nodal analysis software which is available online at petroleum engineering site www.pengtools.com.

Math and Physics

Vogel's IPR equation

$\frac{q_o}{q_{o_{max}}} = 1-0.2 \frac{P_{wf}}{\bar{P}} - 0.8 \left ( \frac{P_{wf}}{\bar{P}} \right )^2$

Darcy's law + Vogel's IPR

Combination single phase liquid constant PI equation and Vogel's IPR:

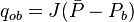$q_{ob} = J (\bar{P} - P_b)$ , oil flow rate at the bubble point.

$q_{o_{max}} = q_{ob} + \frac{J P_b}{1.8}$ , maximum oil rate or absolute open flow (AOF).

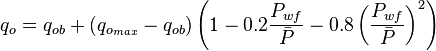$q_o = q_{ob} + (q_{o_{max}} - q_{ob}) \left (1-0.2 \frac{P_{wf}}{\bar{P}} - 0.8 \left ( \frac{P_{wf}}{\bar{P}} \right )^2 \right )$ , oil rate at given flowing bottomhole pressure.

$J = \frac{q_o}{\bar{P}-P_b + \frac{P_b}{1.8} \left (1-0.2 \frac{P_{wf}}{\bar{P}} - 0.8 \left ( \frac{P_{wf}}{\bar{P}} \right )^2 \right ) }$ , productivity index for test below the bubble point pressure.

Discussion

Why Vogel's IPR?

Vogel's IPR solution has been found to be very good and is widely used in prediction of IPR curves.

— Kermit Brown et al

Vogel's IPR calculation workflow

1. Calculate the Productivity Index, J:

1.1 J from the flow test:

• Test is above the bubble point:

$J=\frac{q_{o_{test}}}{\bar{P}-P_{wf}}$

• Test is below the bubble point:

$J = \frac{q_{o_{test}}}{\bar{P}-P_b + \frac{P_b}{1.8} \left (1-0.2 \frac{P_{wf}}{\bar{P}} - 0.8 \left ( \frac{P_{wf}}{\bar{P}} \right )^2 \right ) }$

1.2 J from kh and JD:

$J = \frac{kh\ J_D}{141.2 B \mu}$

1.3 J from kh and skin:

$J = \frac{kh\ \frac{1}{1 / 0.13 + S}}{141.2 B \mu}$

2. Calculate the flowing rates, q_o:

For each P_wf from 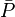$\bar{P}$ to 0:

2.1 Calculate oil flow rate at the bubble point:

$q_{ob} = J (\bar{P} - P_b)$

2.2 Calculate maximum oil rate:

$q_{o_{max}} = q_{ob} + \frac{J P_b}{1.8}$

2.3 Calculate oil rate at given flowing bottomhole pressure, P_wf:

$q_o = q_{ob} + (q_{o_{max}} - q_{ob}) \left (1-0.2 \frac{P_{wf}}{\bar{P}} - 0.8 \left ( \frac{P_{wf}}{\bar{P}} \right )^2 \right )$

Vogel's IPR calculation example

Figure.1 Vogel's IPR calculation example

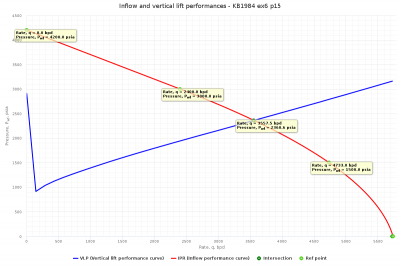
Following the example problem #6, page 15 :

Given:

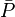$\bar{P}$=4200 psi

J=2 b/d/psi

P_b=3000 psi

Calculate:

(1) q_ob

(2) q_max

(3) q for P_wf=1500 psi

Solution:

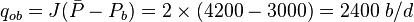
(1) $q_{ob} = J (\bar{P} - P_b) = 2 \times (4200 - 3000) = 2400\ b/d$

(2) $q_{o_{max}} = q_{ob} + \frac{J P_b}{1.8} = 2400 + \frac{2 \times 3000}{1.8} = 2400 + 3333 = 5733\ b/d$

(3 )$q_o = q_{ob} + (q_{o_{max}} - q_{ob}) \left (1-0.2 \frac{P_{wf}}{\bar{P}} - 0.8 \left ( \frac{P_{wf}}{\bar{P}} \right )^2 \right )$

$q_o = 2400 + (5733 - 2400) \left (1-0.2 \frac{1500}{4200} - 0.8 \left ( \frac{1500}{4200} \right )^2 \right )$

$q_o = 2400 + 3333 \times 0.7 = 4733\ b/d$

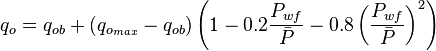
With the help of the PQplot software, other values of flowing pressure has been assumed, and the corresponding values of flow rate were determined. From these, the IPR cure was plotted (Fig.1).

The PQplot model from this example is available online by the following link: Vogel's IPR calculation example

Nomenclature

$B$ = formation volume factor, bbl/stb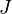

$J$ = productivity index, stb/d/psia

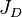$J_D$ = dimensionless productivity index, dimensionless

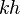$kh$ = permeability times thickness, md*ft

$P$ = pressure, psia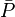

$\bar{P}$ = average reservoir pressure, psia

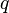$q$ = flowing rate, stb/d

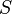$S$ = skin factor, dimensionless

Greek symbols

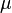$\mu$ = viscosity, cp

Subscripts

b = at bubble point pressure

max = maximum

o = oil

test = well test

wf = well flowing bottomhole pressure

See also

IPR

3 Phase IPR

Darcy's law

JD

141.2 derivation