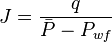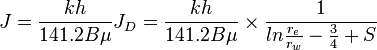Difference between revisions of "Productivity index"

Revision as of 10:30, 14 June 2023

J - well productivity index characterizes how much oil or water the well can produce per unit of pressure drop.

J is defined as follows:

{J} = \frac{q}{\bar{P} - P_{wf}}

From the Darcy's law for an unfractured well located in the center of a circular drainage area, the J in pseudo-steady state is:

{J} = \frac{kh}{141.2 B \mu} {J_D} = \frac{kh}{141.2 B \mu} \times \frac{1}{ln{\frac{r_e}{r_w}-\frac{3}{4}+S}}

Thus, rate could be calculated as:

{q} = \frac{kh}{141.2 B \mu} (\bar{P} - P_{wf}) J_D

The maximum possible stimulated well potential for pseudo steady linear flow is:

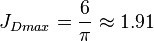${J_D}_{max}= \frac{6}{\pi} \approx 1.91$ , see 6/π stimulated well potential

The maximum possible stimulated well potential for steady state linear flow is:

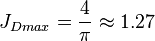${J_D}_{max}= \frac{4}{\pi} \approx 1.27$ , see 4/π stimulated well potential

B

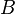= formation volume factor, bbl/stb

J_D

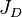= dimensionless productivity index, dimensionless

kh

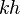= permeability times thickness, md*ft

\bar{P}

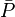= average reservoir pressure, psia

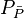P_{\bar{P}}

= average reservoir pseudopressure, psia²/cP

P_{wf}

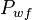= well flowing pressure, psia

P_{P_{wf}}

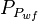= average well flowing pseudopressure, psia²/cP

q

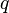= flowing rate, stb/d

q_g

= gas rate, MMscfd

r_w

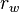= wellbore radius, ft

r_e

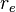= drainage radius, ft

S

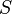= skin factor, dimensionless

T

= temperature, °R

\mu

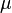= viscosity, cp

@@ Line 9: / Line 9: @@
 drainage area, the [[Productivity index|J]] in pseudo-steady state is:
 :<math> {J} = \frac{kh}{141.2 B \mu} {J_D} = \frac{kh}{141.2 B \mu} \times \frac{1}{ln{\frac{r_e}{r_w}-\frac{3}{4}+S}} </math>
+Thus, rate could be calculated as:
 :<math> {q} = \frac{kh}{141.2 B \mu} (\bar{P} - P_{wf}) J_D</math>