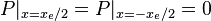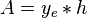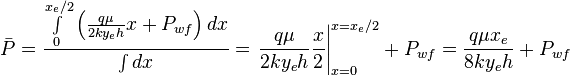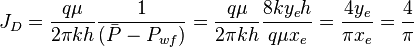Difference between revisions of "6/π stimulated well potential"

From wiki.pengtools.com
Jump to: navigation, search
(→‎Math & Physics)
(→‎Math & Physics)
Line 45: Line 45:
  
 
:<math>\frac{k}{\mu} c \rho \left ( \frac{dP}{dx} \right )^2+ \frac{k \rho}{\mu} \frac{d^2P}{dx^2}=\phi c \rho \frac{dP}{dt}</math> ( 10 )
 
:<math>\frac{k}{\mu} c \rho \left ( \frac{dP}{dx} \right )^2+ \frac{k \rho}{\mu} \frac{d^2P}{dx^2}=\phi c \rho \frac{dP}{dt}</math> ( 10 )
 +
 +
Term <math> \left ( \frac{dP}{dx} \right )^2</math> in (10) is second order of magnitude low and can be cancelled out, which yields:
 +
 +
 +
:<math>\frac{d^2P}{dx^2}=\frac{\phi c \mu}{k} \frac{dP}{dt}</math> ( 11 )
 +
 
----
 
----
 
Integration gives: <math>P-P_{wf}=\frac{q \mu}{2ky_eh} x</math>
 
Integration gives: <math>P-P_{wf}=\frac{q \mu}{2ky_eh} x</math>

Revision as of 09:28, 12 September 2018

Brief

Stimulated well drainage

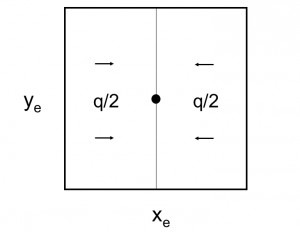
6/π is the maximum possible stimulation potential for pseudo steady state linear flow in a square well spacing.

Math & Physics

Pseudo steady state flow boundary conditions:

P |_{x=x_e/2} = P |_{x=-x_e/2} = 0
\frac{dP}{dt} =const\ for \ \forall x

From Mass conservation:

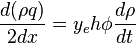\frac{d(\rho q)}{2 dx}=y_e h \phi \frac{d\rho}{dt} ( 1 )

From Darcy's law:

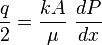\frac{q}{2}=\frac{kA}{\mu}\ \frac{dP}{dx} ( 2 )
A =y_e*h

( 2 ) →( 1 ):

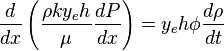\frac{d}{dx} \left ( \frac{\rho k y_e h}{\mu} \frac{dP}{dx} \right )=y_e h \phi \frac{d\rho}{dt} ( 3 )
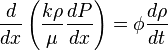\frac{d}{dx} \left ( \frac{k \rho}{\mu} \frac{dP}{dx} \right )=\phi \frac{d\rho}{dt} ( 4 )
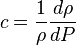c=\frac{1}{\rho} \frac{d \rho}{dP} ( 5 )

( 5 ) -> ( 4 ):

\frac{d}{dx} \left ( \frac{k \rho}{\mu} \frac{dP}{dx} \right )=\phi c \rho \frac{dP}{dt} ( 6 )
\frac{d}{dx} \left ( \frac{k}{\mu} \right ) \rho \frac{dP}{dx} + \frac{k}{\mu} \left ( \frac{d \rho}{dx} \right ) \frac{dP}{dx} + \frac{k \rho}{\mu} \frac{d^2P}{dx^2}=\phi c \rho \frac{dP}{dt} ( 7 )

Assumption that viscosity is constant cancels out first term in left hand side of (7):

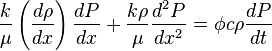\frac{k}{\mu} \left ( \frac{d \rho}{dx} \right ) \frac{dP}{dx} + \frac{k \rho}{\mu} \frac{d^2P}{dx^2}=\phi c \rho \frac{dP}{dt} ( 8 )
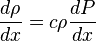\frac{d \rho}{dx} = c \rho \frac{d P}{dx} ( 9 )

( 9 ) -> ( 8 ):

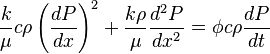\frac{k}{\mu} c \rho \left ( \frac{dP}{dx} \right )^2+ \frac{k \rho}{\mu} \frac{d^2P}{dx^2}=\phi c \rho \frac{dP}{dt} ( 10 )

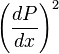Term \left ( \frac{dP}{dx} \right )^2 in (10) is second order of magnitude low and can be cancelled out, which yields:


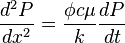\frac{d^2P}{dx^2}=\frac{\phi c \mu}{k} \frac{dP}{dt} ( 11 )

Integration gives: P-P_{wf}=\frac{q \mu}{2ky_eh} x

Since average pressure is: 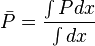\bar P = \frac{\int P dx}{\int dx}

\bar P = \frac{ \int \limits_{0}^{x_e/2} \left ( \frac{q \mu}{2ky_eh} x + P_{wf} \right ) dx}{\int dx} = \left. \frac{q \mu}{2ky_eh} \frac{x}{2} \right|_{x=0}^{x=x_e/2} + P_{wf} = \frac{q \mu x_e}{8ky_eh} + P_{wf}
J_D=\frac{q \mu}{2 \pi k h} \frac{1}{( \bar P - P_{wf})} =\frac{q \mu}{2 \pi k h} \frac{8ky_eh}{q \mu x_e} = \frac{4y_e}{\pi x_e}=\frac{4}{\pi}

See also

optiFrac
fracDesign
Production Potential

Nomenclature

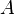A = cross-sectional area, cm2
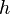h = thickness, m
k = permeability, d
P = pressure, atm
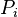P_i = initial pressure, atm
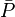\bar P = average pressure, atm
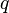q = flow rate, cm3/sec
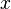x = length, m
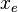x_e = drinage area length, m
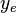y_e = drinage area width, m

Greek symbols

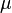\mu = oil viscosity, cp
Retrieved from "https://wiki.pengtools.com/index.php?title=6/π_stimulated_well_potential&oldid=4745"