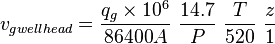Difference between revisions of "Liquid loading"

From wiki.pengtools.com
Jump to: navigation, search
(→‎See also)
 
(12 intermediate revisions by the same user not shown)
Line 57: Line 57:
 
Calculate the gas density:
 
Calculate the gas density:
 
:<math> \rho_g = \frac{28.967\ SG_g\ p}{z\ 10.732\ T_R} = \frac{28.967\ 0.6\ 400}{0.9\ 10.732\ (120+460)}=1.24</math>, lbm/ft2
 
:<math> \rho_g = \frac{28.967\ SG_g\ p}{z\ 10.732\ T_R} = \frac{28.967\ 0.6\ 400}{0.9\ 10.732\ (120+460)}=1.24</math>, lbm/ft2
 +
 +
 +
Calculate the critical gas velocity form (1):
 +
:<math> v_g = 1.593\ \sigma^{1/4}\ \frac{({\rho_L-\rho_g})^{1/4}}{\rho_g^{1/2}} = 1.593\ 60^{1/4}\ \frac{({67-1.24})^{1/4}}{1.24^{1/2}}=11.3</math>, ft/sec
 +
 +
 +
Calculate the critical gas rate form (2):
 +
:<math> q_g = 3.067\ \frac{P\ v_g\ A}{T\ z} = 3.067\ \frac{400\ 11.3\ 0.0217}{(120+460)\ 0.9}=0.578 </math>, MMscfd
 +
 +
===Solution with PQplot===
 +
[[PQplot]] calculates the following critical gas rate:
 +
:<math> q_g = 0.56 </math>, MMscfd
 +
 +
[[File:Liquid loading example 3-1.png|1000px| Liquid loading example 3-1]]<br>
 +
[https://www.pengtools.com/pqPlot?paramsToken=c97c4f3b499cf59b82d61c374d3ab21a Liquid loading example 3-1 (Public model)]
  
 
== Nomenclature ==
 
== Nomenclature ==
Line 65: Line 80:
 
:<math> \rho_L </math> = liquid density, lbm/ft3
 
:<math> \rho_L </math> = liquid density, lbm/ft3
 
:<math> \sigma </math> = surface tension, dyne/cm (ref values: 60 - water, 20 - condensate) <ref name=Turner/>
 
:<math> \sigma </math> = surface tension, dyne/cm (ref values: 60 - water, 20 - condensate) <ref name=Turner/>
 +
:<math> SG_g </math> = gas specific gravity
 
:<math> T </math> = flowing wellhead temperature, °R
 
:<math> T </math> = flowing wellhead temperature, °R
 
:<math> v_g </math> = gas velocity, ft/sec
 
:<math> v_g </math> = gas velocity, ft/sec
 
:<math> z </math> = gas compressibility factor at flowing P & T, dimensionless
 
:<math> z </math> = gas compressibility factor at flowing P & T, dimensionless
 +
 +
== See also ==
 +
[[Gray|Gray correlation]]
  
 
== References ==
 
== References ==

Latest revision as of 10:45, 8 May 2024

Brief

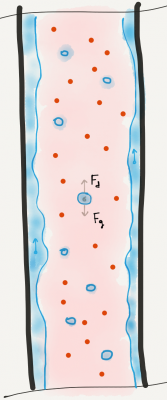
Liquid loading is a phenomenon when the gas phase does't provide sufficient transport energy to lift the liquids out of the well.

In 1969 Turner et al. published an empirical correlation defining the Liquid loading gas velocity[1].

Liquid Loading

Math & Physics

The minimum gas velocity to remove the liquid equation:

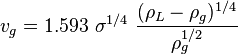v_g = 1.593\ \sigma^{1/4}\ \frac{({\rho_L-\rho_g})^{1/4}}{\rho_g^{1/2}}[2] (1)

Note that original paper[1] uses 20.4 constant which is good if the surface tension units are in 'lbf/ft'. Also 20.4 is calculated by adjusting the theoretical equation constant by 20 percent. For the reference PROSPER uses 2.04. PQplot uses 1.593.

The minimum gas rate to remove the liquid equation:

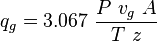q_g = 3.067\ \frac{P\ v_g\ A}{T\ z} (2)

PQplot workflow

In order to calculate the liquid loading point in PQplot:
For every point in the VLP curve:
1. Critical gas velocity at the wellhead is calculated by eq (1)
2. Wellhead flowing gas velocity is calculated

v_{g wellhead} = \frac{q_g \times 10^6}{86400 A}\ \frac{14.7}{P}\ \frac{T}{520}\ \frac{z}{1}

3. Liquid loading flag is 1 if:

v_{g wellhead} < v_{g critical}

4. Critical gas rate is the last VLP curve point at which liquid loading flag is 1.

Discussion

To avoid the Liquid loading the gas velocity should be above the Liquid loading velocity.

The higher the gas rate the higher the gas velocity.

The lower the wellhead flowing pressure the higher the gas rate.

The bigger the tubing ID the higher the gas rate.

In case when the gas rate is limited by the Reservoir deliverability smaller tubing ID will increase the gas velocity.

Example. Calculating critical gas velocity and rate

Example 3-2 from [2]

Input data

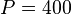P = 400 , flowing wellhead pressure, psia
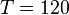T =120 , flowing wellhead temperature, °F
\rho_L =67, liquid density, lbm/ft3
\sigma = 60 , water surface tension, dyne/cm
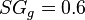SG_g = 0.6 , gas specific gravity
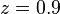z =0.9 , gas compressibility factor

Production string = 2-3/8 inch tubing with 1.995 in ID, A = .0217 ft2
Water is the produced liquid

Solution

Calculate the gas density:

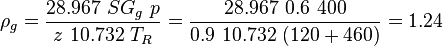\rho_g = \frac{28.967\ SG_g\ p}{z\ 10.732\ T_R} = \frac{28.967\ 0.6\ 400}{0.9\ 10.732\ (120+460)}=1.24, lbm/ft2


Calculate the critical gas velocity form (1):

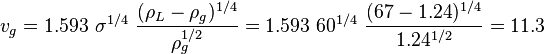v_g = 1.593\ \sigma^{1/4}\ \frac{({\rho_L-\rho_g})^{1/4}}{\rho_g^{1/2}} = 1.593\ 60^{1/4}\ \frac{({67-1.24})^{1/4}}{1.24^{1/2}}=11.3, ft/sec


Calculate the critical gas rate form (2):

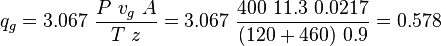q_g = 3.067\ \frac{P\ v_g\ A}{T\ z} = 3.067\ \frac{400\ 11.3\ 0.0217}{(120+460)\ 0.9}=0.578 , MMscfd

Solution with PQplot

PQplot calculates the following critical gas rate:

q_g = 0.56 , MMscfd

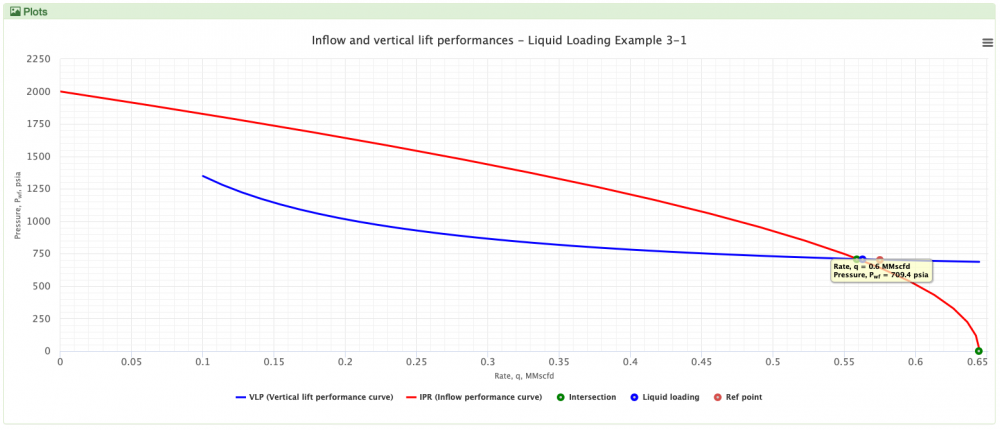
Liquid loading example 3-1
Liquid loading example 3-1 (Public model)

Nomenclature

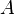A = flow area, ft^2
P = flowing wellhead pressure, psia
q_g = gas rate, MMscf/d
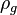\rho_g = gas density, lbm/ft3
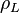\rho_L = liquid density, lbm/ft3
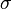\sigma = surface tension, dyne/cm (ref values: 60 - water, 20 - condensate) [1]
SG_g = gas specific gravity
T = flowing wellhead temperature, °R
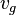v_g = gas velocity, ft/sec
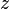z = gas compressibility factor at flowing P & T, dimensionless

See also

Gray correlation

References

  1. ↑ 1.0 1.1 1.2 Turner, R. G.; Hubbard, A. E.; Dukler (Nov 1969). "Analysis and Prediction of Minimum Flow Rate for the Continuous Removal of Liquids from Gas Wells"Free registration required. Journal of Petroleum Technology (SPE-2198-PA): 1475–1482. 
  2. ↑ 2.0 2.1 Lea, J. F.; Nickens, M.; Wells (2011). Gas Well DeliquificationPaid subscription required. Energy Tribune Publishing Inc. 
Retrieved from "https://wiki.pengtools.com/index.php?title=Liquid_loading&oldid=7788"