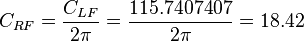Difference between revisions of "18.41 derivation"
From wiki.pengtools.com
(Created page with "== Brief== '''18.41''' is the well know constant which is used for converting from the Darcy's law units to the metric units in the well's inflow equations. For example...") |
|||
| (13 intermediate revisions by the same user not shown) | |||
| Line 3: | Line 3: | ||
'''18.41''' is the well know constant which is used for converting from the [[Darcy's law]] units to the metric units in the well's inflow equations. | '''18.41''' is the well know constant which is used for converting from the [[Darcy's law]] units to the metric units in the well's inflow equations. | ||
| − | For example [[Darcy's law]] for the single-phase flow is as follows<ref name= | + | For example [[Darcy's law]] for the single-phase flow is as follows<ref name=DW/>: |
:<math> q_o = \frac{1}{18.41} \times \frac{k_oh}{B_o\mu_o} \times \Delta P \times J_D</math> | :<math> q_o = \frac{1}{18.41} \times \frac{k_oh}{B_o\mu_o} \times \Delta P \times J_D</math> | ||
| − | The derivation of the | + | The derivation of the 18.41 constant is given below. |
== Math and Physics == | == Math and Physics == | ||
| Line 20: | Line 20: | ||
:<math> \frac{[cm^3]}{[sec]} = - \frac{[D][cm^2]}{[cP]} \frac{[atm]}{[cm]}</math> | :<math> \frac{[cm^3]}{[sec]} = - \frac{[D][cm^2]}{[cP]} \frac{[atm]}{[cm]}</math> | ||
| − | Converting to the | + | Converting to the metric units: |
| − | :<math> \frac{[cm^3] \frac{[ | + | :<math> \frac{[cm^3] \frac{[m^3]}{[1000000 cm^3]}}{[sec] \frac{[day]}{[86400 sec]}} = - \frac{[D] \frac{[1000 mD]}{[D]}[cm^2] \frac{[m^2]}{[10000 cm^2]}}{[cP]} \frac{[atm]}{[cm] \frac{[m]}{[100 cm]}}</math> |
So: | So: | ||
| − | :<math> \frac{[ | + | :<math> \frac{[m^3]}{[day]} \frac{86400}{1000000} = - \frac{[mD][m^2]}{[cP]} \frac{[atm]}{[m]} \frac{1000 \times 100}{10000}</math> |
And: | And: | ||
| − | :<math> \frac{[ | + | :<math> \frac{[m^3]}{[day]} = - C_{LF} \frac{[mD][m^2]}{[cP]} \frac{[atm]}{[m]}</math> |
where | where | ||
| − | :<math> C_{LF} = \frac{1000\ | + | :<math> C_{LF} = \frac{1000 \times 100}{10000} \frac{1000000}{86400} = \frac{10000000}{86400} = 115.7407407</math> |
For the radial flow: | For the radial flow: | ||
| − | :<math> C_{RF} = \frac{C_{LF}}{2\pi} = \frac{ | + | :<math> C_{RF} = \frac{C_{LF}}{2\pi} = \frac{115.7407407}{2\pi} = 18.42 </math> |
| − | + | Note that the published<ref name=DW/> and commonly used constant in '''18.41'''. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
== Nomenclature == | == Nomenclature == | ||
:<math> A </math> = [[Darcy's law]] cross-sectional area, cm<sup>2</sup> | :<math> A </math> = [[Darcy's law]] cross-sectional area, cm<sup>2</sup> | ||
| − | :<math> B_o </math> = oil formation volume factor, | + | :<math> B_o </math> = oil formation volume factor, m^3/m^3 |
:<math> C_{LF} </math> = linear flow units conversion constant | :<math> C_{LF} </math> = linear flow units conversion constant | ||
:<math> C_{RF} </math> = radial flow units conversion constant | :<math> C_{RF} </math> = radial flow units conversion constant | ||
| − | :<math> h</math> = effective feet of oil pay, | + | :<math> h</math> = effective feet of oil pay, m |
:<math> J_D </math> = dimensionless productivity index, dimensionless | :<math> J_D </math> = dimensionless productivity index, dimensionless | ||
:<math> k</math> = [[Darcy's law]] permeability, d | :<math> k</math> = [[Darcy's law]] permeability, d | ||
| Line 61: | Line 54: | ||
:<math> L </math> = [[Darcy's law]] length, cm | :<math> L </math> = [[Darcy's law]] length, cm | ||
:<math> P </math> = [[Darcy's law]] pressure, atm | :<math> P </math> = [[Darcy's law]] pressure, atm | ||
| − | :<math> \Delta P </math> = drawdown, | + | :<math> \Delta P </math> = drawdown, atm |
:<math> q </math> = [[Darcy's law]] flow rate, cm<sup>3</sup>/sec | :<math> q </math> = [[Darcy's law]] flow rate, cm<sup>3</sup>/sec | ||
| − | :<math> q_o </math> = oil flow rate, | + | :<math> q_o </math> = oil flow rate, m^3/d |
===Greek symbols=== | ===Greek symbols=== | ||
| Line 69: | Line 62: | ||
:<math> \mu </math> = [[Darcy's law]] oil viscosity, cp | :<math> \mu </math> = [[Darcy's law]] oil viscosity, cp | ||
:<math> \mu_o </math> = oil viscosity, cp | :<math> \mu_o </math> = oil viscosity, cp | ||
| + | |||
| + | ==See Also== | ||
| + | [[Darcy's law]]<BR/> | ||
| + | [[141.2 derivation]]<BR/> | ||
| + | [[18.41 derivation]] | ||
==References== | ==References== | ||
<references> | <references> | ||
| − | + | <ref name=DW> | |
| − | <ref name= | + | {{cite book |
| − | |last1= | + | |last1= Wolcott |first1=Don |
| − | |title= | + | |title=Applied Waterflood Field Development |
| − | |publisher= | + | |date=2009 |
| − | | | + | |publisher=Energy Tribune Publishing Inc |
| − | | | + | |place=Houston |
| − | | | + | |url=https://www.amazon.com/Applied-Waterflood-Field-Development-Wolcott/dp/0578023946/ref=sr_1_1?ie=UTF8&qid=1481788841&sr=8-1&keywords=Don+wolcott |
| + | |url-access=subscription | ||
}}</ref> | }}</ref> | ||
| − | |||
</references> | </references> | ||
| Line 94: | Line 92: | ||
|description=18.41 is the well know constant which is used for converting from the Darcy's law units to the metric units in the well's inflow equations. | |description=18.41 is the well know constant which is used for converting from the Darcy's law units to the metric units in the well's inflow equations. | ||
}} | }} | ||
| + | <div style='text-align: right;'>By Mikhail Tuzovskiy on {{REVISIONTIMESTAMP}}</div> | ||
Latest revision as of 12:32, 12 July 2023
Brief
18.41 is the well know constant which is used for converting from the Darcy's law units to the metric units in the well's inflow equations.
For example Darcy's law for the single-phase flow is as follows[1]:
The derivation of the 18.41 constant is given below.
Math and Physics
In Darcy's units:
Converting to the metric units:
So:
And:
where
For the radial flow:
Note that the published[1] and commonly used constant in 18.41.
Nomenclature
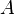 = Darcy's law cross-sectional area, cm2
= Darcy's law cross-sectional area, cm2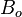 = oil formation volume factor, m^3/m^3
= oil formation volume factor, m^3/m^3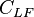 = linear flow units conversion constant
= linear flow units conversion constant 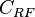 = radial flow units conversion constant
= radial flow units conversion constant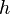 = effective feet of oil pay, m
= effective feet of oil pay, m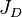 = dimensionless productivity index, dimensionless
= dimensionless productivity index, dimensionless = Darcy's law permeability, d
= Darcy's law permeability, d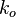 = effective permeability to oil, md
= effective permeability to oil, md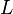 = Darcy's law length, cm
= Darcy's law length, cm = Darcy's law pressure, atm
= Darcy's law pressure, atm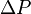 = drawdown, atm
= drawdown, atm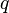 = Darcy's law flow rate, cm3/sec
= Darcy's law flow rate, cm3/sec = oil flow rate, m^3/d
= oil flow rate, m^3/d
Greek symbols
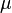 = Darcy's law oil viscosity, cp
= Darcy's law oil viscosity, cp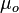 = oil viscosity, cp
= oil viscosity, cp
See Also
Darcy's law
141.2 derivation
18.41 derivation
References
- ↑ 1.0 1.1
Wolcott, Don (2009). Applied Waterflood Field Development
 . Houston: Energy Tribune Publishing Inc.
. Houston: Energy Tribune Publishing Inc.
By Mikhail Tuzovskiy on 20230712123245
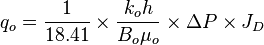

![\frac{[cm^3]}{[sec]} = - \frac{[D][cm^2]}{[cP]} \frac{[atm]}{[cm]}](/images/math/5/5/5/555b9dc7f65e6db7f1cd05153aaae770.png)
![\frac{[cm^3] \frac{[m^3]}{[1000000 cm^3]}}{[sec] \frac{[day]}{[86400 sec]}} = - \frac{[D] \frac{[1000 mD]}{[D]}[cm^2] \frac{[m^2]}{[10000 cm^2]}}{[cP]} \frac{[atm]}{[cm] \frac{[m]}{[100 cm]}}](/images/math/a/f/f/aff7f8a69e17996a0603dfdb3d10146d.png)
![\frac{[m^3]}{[day]} \frac{86400}{1000000} = - \frac{[mD][m^2]}{[cP]} \frac{[atm]}{[m]} \frac{1000 \times 100}{10000}](/images/math/1/9/a/19a0f2857c17986a841f1a1aef5d6fc0.png)
![\frac{[m^3]}{[day]} = - C_{LF} \frac{[mD][m^2]}{[cP]} \frac{[atm]}{[m]}](/images/math/9/4/b/94bc5860b563656c7f4601c8fb7b4b52.png)