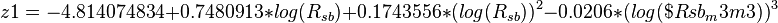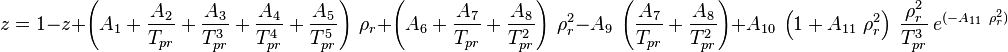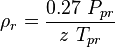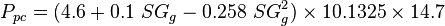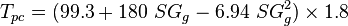Difference between revisions of "Valko - McCain bubble point pressure correlation"

From wiki.pengtools.com
Jump to: navigation, search
(Created page with "__TOC__ === Brief === Valco- McCain correlation is ... === Math & Physics === :<math> z1 = -4.814074834 + 0.7480913 * log(R_{sb}) + 0.1743556 * (log(R_{sb}))^2 - 0.0206...")
(No difference)

Revision as of 08:34, 13 June 2017

Brief

Valco- McCain correlation is ...

Math & Physics

z1 = -4.814074834 + 0.7480913 * log(R_{sb}) + 0.1743556 * (log(R_{sb}))^2 - 0.0206 * (log($Rsb_m3m3))^3

z2 = 1.27 - 0.0449 * $API + 0.000436 * pow($API, 2) - 0.00000476 * pow($API, 3); z3 = 4.51 - 10.84 * $sgGas + 8.39 * pow($sgGas, 2) - 2.34 * pow($sgGas, 3); z4 = -7.2254661 + 0.043155 * $tKel - 8.5548e-5 * pow($tKel, 2) + 6.00696e-8 * pow($tKel, 3); z = $z1 + $z2 + $z3 + $z4;

lnPb = 2.498 + 0.713 * $z + 0.0075 * pow($z, 2);

Bubblepoint_Valko_McCainSI = exp($lnPb);

z = 1-z+ \left(A_1 +\frac{A_2}{T_{pr}} +\frac{A_3}{T^3_{pr}} +\frac{A_4}{T^4_{pr}} +\frac{A_5}{T^5_{pr}} \right)\ \rho_r+ \left(A_6 +\frac{A_7}{T_{pr}} +\frac{A_8}{T^2_{pr}} \right)\ \rho^2_r -A_9\ \left(\frac{A_7}{T_{pr}}+\frac{A_8}{T^2_{pr}}\right) +A_{10}\ \left(1+A_{11}\ \rho^2_r\right)\ \frac{\rho^2_r}{T^3_{pr}} \ e^{(-A_{11}\ \rho^2_r)} [1]

where:

\rho_r = \frac{0.27\ P_{pr}}{{z\ T_{pr}}}
P_{pr} = \frac{P}{P_{pc}}
T_{pr} = \frac{T}{T_{pc}}

Discussion

Why the Dranchuk correlation?

It's classics!
— www.pengtools.com

Workflow

To solve the Dranchuk equation use the iterative secant method.

To find the pseudo critical properties from the gas specific gravity [2]:

P_{pc} = ( 4.6+0.1\ SG_g-0.258\ SG^2_g ) \times 10.1325 \times 14.7
T_{pc} = ( 99.3+180\ SG_g-6.94\ SG^2_g ) \times 1.8

Application range

0.2 \le P_{pr} < 30 ; 1.0 < T_{pr} \le 3.0 [1]

and

P_{pr} < 1.0 ; 0.7 < T_{pr} \le 1.0[1]

Nomenclature

A_1..A_{11} = coefficients
\rho_r = reduced density, dimensionless
P = pressure, psia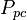
P_{pc} = pseudo critical pressure, psia
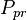P_{pr} = pseudoreduced pressure, dimensionless
SG_g = gas specific gravity, dimensionless
T = temperature, °R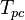
T_{pc} = pseudo critical temperature, °R
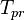T_{pr} = pseudoreduced temperature, dimensionless
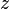z = gas compressibility factor, dimensionless

References

  1. ↑ 1.0 1.1 1.2 Dranchuk, P. M.; Abou-Kassem, H. (July 1975). "Calculation of Z Factors For Natural Gases Using Equations of State"Free registration required. The Journal of Canadian Petroleum. 14 (PETSOC-75-03-03). 
  2. ↑ Standing, M. B.; Katz, D. L. (December 1942). "Density of Natural Gases"Free registration required. Transactions of the AIME. Society of Petroleum Engineers. 146 (SPE-942140-G). 
Retrieved from "https://wiki.pengtools.com/index.php?title=Valko_-_McCain_bubble_point_pressure_correlation&oldid=2527"