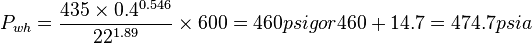Difference between revisions of "Gilbert choke equation"
From wiki.pengtools.com
(→Solution) |
|||
| Line 17: | Line 17: | ||
===Solution=== | ===Solution=== | ||
| − | :<math>P_{wh}=\frac{435 \times 0.4^{0.546}}{22^{1.89}} \times 600 = 460 psig | + | :<math>P_{wh}=\frac{435 \times 0.4^{0.546}}{22^{1.89}} \times 600 = 460 psig or 460 +14.7 = 474.7 psia</math> |
Validity check 180/460=0.4 < 0.7 OK | Validity check 180/460=0.4 < 0.7 OK | ||
Revision as of 18:58, 8 November 2024
Contents
Brief
The most common formula used for multiphase flow through surface chokes by Gilbert [1][2].
Gilbert developed his empirical equation from field data in California.
Math and Physics
Note that the equation is independent of the downstream pressure and assumes that the downstream pressure is less than 70% of the upstream pressure, i.e. the flow is "critical" and fluid reach sonic velocity in the throat of the choke.
Example
Given data
Oil rate = 600 bbl/d, GLR=400 scf/bbl, D=22/64 in, Line pressure = 180 psia
Calculate the well head pressure?
Solution
Validity check 180/460=0.4 < 0.7 OK
Nomenclature
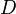= choke beam diametr, 64th of an inch
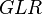= gas liquid ratio, Mscf/bbl or 10^3 scf/bbl
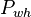= well head pressure, psig
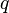= flow rate, bbl/d
References
