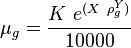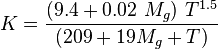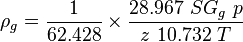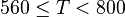Difference between revisions of "Lee correlation"
From wiki.pengtools.com
| Line 1: | Line 1: | ||
__TOC__ | __TOC__ | ||
| − | === | + | === Lee gas viscosity correlation === |
[[Lee correlation]] is the empirical correlation for the gas viscosity published in '''1966'''. | [[Lee correlation]] is the empirical correlation for the gas viscosity published in '''1966'''. | ||
| Line 58: | Line 58: | ||
{{#seo: | {{#seo: | ||
| − | |title=Lee | + | |title=Lee gas viscosity correlation |
|titlemode= replace | |titlemode= replace | ||
|keywords=gas viscosity, Lee correlation | |keywords=gas viscosity, Lee correlation | ||
|description=Lee correlation is the empirical correlation for the gas viscosity published in 1966. | |description=Lee correlation is the empirical correlation for the gas viscosity published in 1966. | ||
}} | }} | ||
Revision as of 11:55, 5 October 2020
Contents
Lee gas viscosity correlation
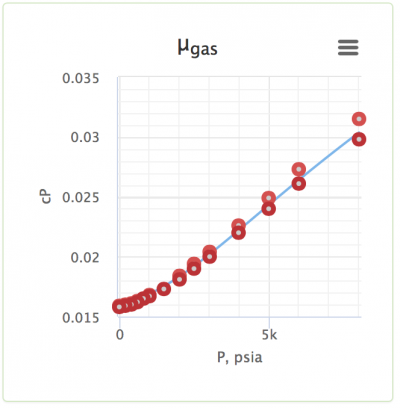
Lee correlation is the empirical correlation for the gas viscosity published in 1966.
Math & Physics
where
Discussion
Why the Lee correlation?
Application range
Nomenclature
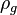= gas density, g/cm3
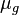= gas viscosity, cp
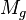= gas molecular weight, dimensionless
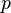= pressure, psia
= gas specific gravity, dimensionless
= temperature, °R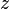
= gas compressibility factor, dimensionless
References
- ↑ Lee, A. B.; Gonzalez, M. H.; Eakin, B. E. (1966). "The Viscosity of Natural Gases". Journal of Petroleum Technology (SPE-1340-PA).
