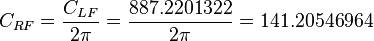Difference between revisions of "141.2 derivation"
From wiki.pengtools.com
| Line 3: | Line 3: | ||
'''141.2''' is the well know constant which is used for converting from the Darcy's law units to the field units in the well's inflow equations. | '''141.2''' is the well know constant which is used for converting from the Darcy's law units to the field units in the well's inflow equations. | ||
| − | For example Darcy's law for the single-phase flow is as follows: | + | For example Darcy's law for the single-phase flow is as follows<ref name=KermitBrown/>: |
:<math> q_o = \frac{1}{141.2} \times \frac{k_oh}{B_o\mu_o} \times \Delta P \times J_D = 7.08 \times 10^-3 \times \frac{k_oh}{B_o\mu_o} \times \Delta P \times J_D</math> | :<math> q_o = \frac{1}{141.2} \times \frac{k_oh}{B_o\mu_o} \times \Delta P \times J_D = 7.08 \times 10^-3 \times \frac{k_oh}{B_o\mu_o} \times \Delta P \times J_D</math> | ||
| Line 49: | Line 49: | ||
18.41 derivation | 18.41 derivation | ||
| − | + | <references> | |
| + | <ref name=KermitBrown>{{cite book | ||
| + | |last1=Brown|first1= Kermit | ||
| + | |title=The Technology of Artificial Lift Methods. Volume 4. Production Optimization of Oil and Gas Wells by Nodal System Analysis | ||
| + | |publisher=PennWellBooks | ||
| + | |volume=2 | ||
| + | |date=1984 | ||
| + | |place=Tulsa, Oklahoma | ||
| + | }}</ref> | ||
| + | |||
| + | |||
| + | </references> | ||
[[Category:Technology]] | [[Category:Technology]] | ||
Revision as of 05:55, 23 April 2018
Brief
141.2 is the well know constant which is used for converting from the Darcy's law units to the field units in the well's inflow equations.
For example Darcy's law for the single-phase flow is as follows[1]:
The derivation of the 141.2 constant is given below.
Math and Physics
Darcy's law:
In Darcy's units:
Converting to the field units:
So:
And:
where
For the radial flow:
One can be familiar with the inverse of the 141.2 constant:
See Also
Darcy's law
18.41 derivation
- ↑ Brown, Kermit (1984). The Technology of Artificial Lift Methods. Volume 4. Production Optimization of Oil and Gas Wells by Nodal System Analysis. 2. Tulsa, Oklahoma: PennWellBooks.
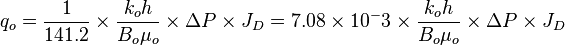

![\frac{[cm^3]}{[sec]} = - \frac{[D][cm^2]}{[cP]} \frac{[atm]}{[cm]}](/images/math/5/5/5/555b9dc7f65e6db7f1cd05153aaae770.png)
![\frac{[cm^3] \frac{[ft^3]}{[30.48^3 cm^3]} \frac{[bbl]}{[5.61458333 ft^3]} }{[sec] \frac{[day]}{[86400 sec]}} = - \frac{[D] \frac{[1000 mD]}{[D]}[cm^2] \frac{[ft^2]}{[30.48^2 cm]}}{[cP]} \frac{[atm] \frac{[14.695950253959 psia]}{[atm]}}{[cm] \frac{[ft]}{[30.48 cm]}}](/images/math/1/9/0/190503f228c14db727074159d40e455b.png)
![\frac{[bbl]}{[day]} \frac{86400}{30.48^3\ 5.61458333} = - \frac{[mD][ft^2]}{[cP]} \frac{[psia]}{[ft]} \frac{1000\ 14.695950253959}{30.48}](/images/math/9/a/b/9abe955d3e8338add13d1fe96e9fd738.png)
![\frac{[bbl]}{[day]} = - C_{LF} \frac{[mD][ft^2]}{[cP]} \frac{[psia]}{[ft]}](/images/math/d/d/4/dd4c5bbb71e5c38a6ae238f62d56d9ea.png)