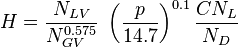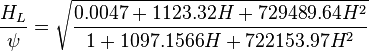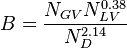Difference between revisions of "Hagedorn and Brown correlation"
From wiki.pengtools.com
(→Nomenclature) |
(→Nomenclature) |
||
| Line 67: | Line 67: | ||
:<math> \psi = \begin{cases} | :<math> \psi = \begin{cases} | ||
27170 B^3 - 317.52 B^2 + 0.5472 B + 0.9999, & \mbox{if }B\mbox{ = 0.025} \\ | 27170 B^3 - 317.52 B^2 + 0.5472 B + 0.9999, & \mbox{if }B\mbox{ = 0.025} \\ | ||
| − | -533.33 B^2 + 58.524 B + 0.1171, & \mbox{if }B\mbox{ 0.025} \\ | + | -533.33 B^2 + 58.524 B + 0.1171, & \mbox{if }B\mbox{ \< 0.025} \\ |
2.5714 B +1.5962, & \mbox{if }B\mbox{ 0.055} | 2.5714 B +1.5962, & \mbox{if }B\mbox{ 0.055} | ||
\end{cases} </math> | \end{cases} </math> | ||
Revision as of 13:17, 21 March 2017
Contents
Brief
Hagedorn and Brown is an empirical two-phase flow correlation published in 1965.
It doesn't distinguish between the flow regimes.
The heart of the Hagedorn and Brown method is a correlation for the liquid holdup : .
.
Math & Physics
Following the law of conservation of energy the basic steady state flow equation is:
where
Colebrook–White equation for the Darcy's friction factor:
Reynolds two phase number:
Discussion
Block Diagram
Workflow
- Failed to parse (PNG conversion failed; check for correct installation of latex and dvipng (or dvips + gs + convert)): \psi = \begin{cases} 27170 B^3 - 317.52 B^2 + 0.5472 B + 0.9999, & \mbox{if }B\mbox{ 0.025} \\ -533.33 B^2 + 58.524 B + 0.1171, & \mbox{if }B\mbox{ 0.025} 2.5714 B +1.5962, & \mbox{if }B\mbox{ 0.055} \end{cases}
Nomenclature
- Failed to parse (lexing error): \psi = \begin{cases} 27170 B^3 - 317.52 B^2 + 0.5472 B + 0.9999, & \mbox{if }B\mbox{ = 0.025} \\ -533.33 B^2 + 58.524 B + 0.1171, & \mbox{if }B\mbox{ \< 0.025} \\ 2.5714 B +1.5962, & \mbox{if }B\mbox{ 0.055} \end{cases}
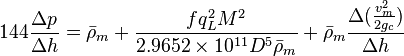
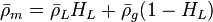
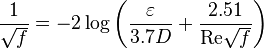
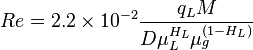
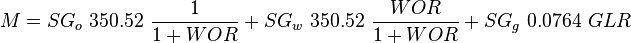
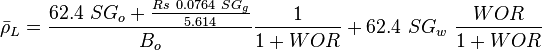
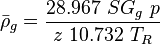

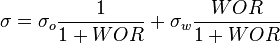
![N_L = 0.15726\ \mu_L \sqrt[4]{\frac{1}{\rho_L \sigma_L^3}}](/images/math/b/2/0/b207fe79b4a4ee53d466e182791ca737.png)
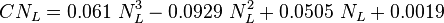

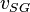
![N_{LV} = 1.938\ v_{SL}\ \sqrt[4]{\frac{\rho_L}{\sigma}}](/images/math/2/f/2/2f2abb2b5e504663beb5ddb87301af09.png)
![N_{GV} = 1.938\ v_{SG}\ \sqrt[4]{\frac{\rho_L}{\sigma}}](/images/math/4/0/c/40cab20a6f3a6a92f320bbff38c696cd.png)