Difference between revisions of "Darcy's law"
From wiki.pengtools.com
(→Darcy's law) |
(→Darcy's law History) |
||
| Line 9: | Line 9: | ||
== Darcy's law History == | == Darcy's law History == | ||
| − | [[Darcy's law]] is the fundamental '''law''' of fluid motion in porous media published by '''Henry Darcy''' | + | [[Darcy's law]] is the fundamental '''law''' of fluid motion in porous media published by '''Henry Darcy''' In '''1856''' <ref name=DakeF/>. |
| + | |||
| + | In '''1856''' '''Henry Darcy''' published a detailed account of his work on the design of a filter large enough to process the towns daily water requirement <ref name=DakeF/>. | ||
[[File:Les Fontaines Publiques de la Ville de Dijon.png|200px |link=https://books.google.ru/books?id=-FxYAAAAYAAJ&printsec=frontcover&hl=ru&source=gbs_ge_summary_r&cad=0#v=twopage&q&f=false]] | [[File:Les Fontaines Publiques de la Ville de Dijon.png|200px |link=https://books.google.ru/books?id=-FxYAAAAYAAJ&printsec=frontcover&hl=ru&source=gbs_ge_summary_r&cad=0#v=twopage&q&f=false]] | ||
Revision as of 13:46, 22 July 2019
Contents
Darcy's law
Darcy's law is the fundamental law of fluid motion in porous media published by Henry Darcy in 1856 [1].
Darcy's law has been applied successfully to determine the flow through permeable media since the early days of Petroleum Engineering.
Darcy's law History
Darcy's law is the fundamental law of fluid motion in porous media published by Henry Darcy In 1856 [2].
In 1856 Henry Darcy published a detailed account of his work on the design of a filter large enough to process the towns daily water requirement [2].
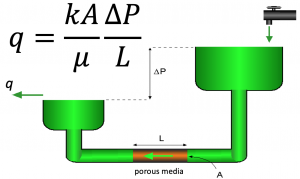
Darcy's law Equation
Conditions
- Single fluid
- Steady stay flow
- Constant fluid compressibility
- Constant temperature
Inflow Equations Derivation
Derivation of the Linear and Radial Inflow Equations
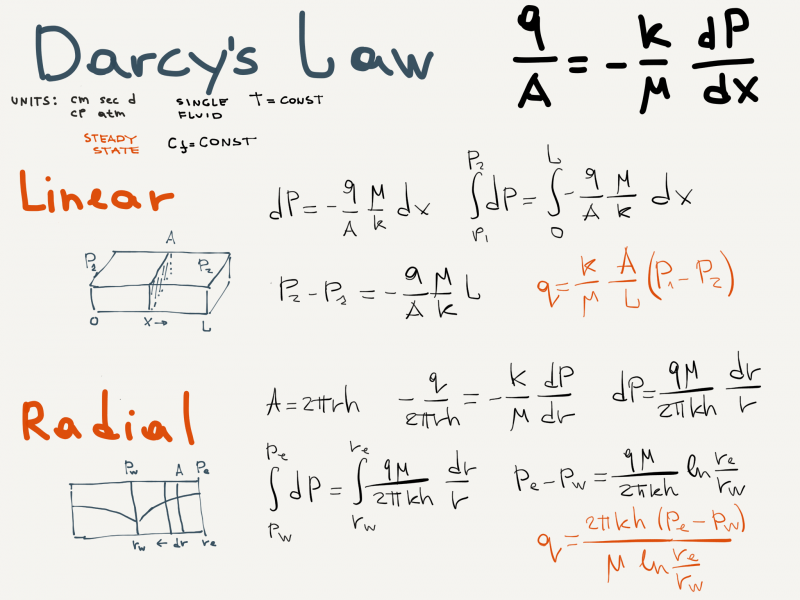
Nomenclature
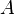 = cross-sectional area, cm2
= cross-sectional area, cm2 = permeability, d
= permeability, d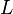 = length, cm
= length, cm = pressure, atm
= pressure, atm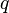 = flow rate, cm3/sec
= flow rate, cm3/sec
Greek symbols
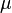 = Darcy's law fluid viscosity, cp
= Darcy's law fluid viscosity, cp
See Also
Darcy's law application in Petroleum Engineering Technology.
References
- ↑ Darcy, Henry (1856). "Les Fontaines Publiques de la Ville de Dijon". Paris: Victor Dalmont.
- ↑ 2.0 2.1 Dake, L.P. (1978). Fundamentals of Reservoir Engineering. Amsterdam, Hetherlands: Elsevier Science. ISBN 0-444-41830-X.