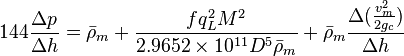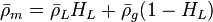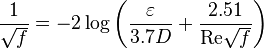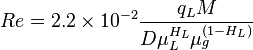Difference between revisions of "Hagedorn and Brown correlation"

Revision as of 09:37, 16 March 2017

Hagedorn and Brown is an empirical two-phase flow correlation published in 1965.

It doesn't distinguish between the flow regimes.

The heart of the Hagedorn and Brown method is a correlation for the liquid holdup : $H_L$ .

Following the law of conservation of energy the basic steady state flow equation is:

144 \frac{\Delta p}{\Delta h} = \bar \rho_m + \frac{f q_L^2 M^2}{2.9652 \times 10^{11} D^5 \bar \rho_m} + \bar \rho_m \frac{\Delta{(\frac{v_m^2}{2g_c}})}{\Delta h}

where

\bar \rho_m = \bar \rho_L H_L + \bar \rho_g (1 - H_L)

Colebrook–White equation for the Darcy's friction factor:

\frac{1}{\sqrt{f}}= -2 \log \left( \frac { \varepsilon} {3.7 D} + \frac {2.51} {\mathrm{Re} \sqrt{f}} \right)

Reynolds two phase number:

Re = 2.2 \times 10^{-2} \frac {q_L M}{D \mu_L^{H_L} \mu_g^{(1-H_L)}}

For each pipe segment find:

@@ Line 8: / Line 8: @@
 == Math & Physics ==
-The basic steady state flow equation is:
+Following the law of conservation of energy the basic steady state flow equation is:
 :<math> 144 \frac{\Delta p}{\Delta h} = \bar \rho_m + \frac{f q_L^2 M^2}{2.9652 \times 10^{11} D^5 \bar \rho_m} + \bar \rho_m \frac{\Delta{(\frac{v_m^2}{2g_c}})}{\Delta h}</math>
 where