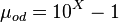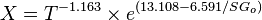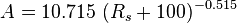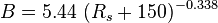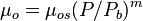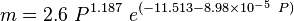Difference between revisions of "Beggs and Robinson Oil Viscosity correlation"
From wiki.pengtools.com
| Line 27: | Line 27: | ||
Undersaturated oil viscosity: | Undersaturated oil viscosity: | ||
| − | :<math>\mu_{o} = \mu_{os} (P/P_b)^m </math><ref name=VB1980> | + | :<math>\mu_{o} = \mu_{os} (P/P_b)^m </math><ref name=VB1980/> |
where: | where: | ||
Revision as of 10:05, 25 July 2017
Brief
Beggs - Robinson is an empirical correlation for the oil viscosity published in 1975 [1].
Math & Physics
Dead oil viscosity:
where:
Saturated oil viscosity:
where:
Undersaturated oil viscosity:
where:
Discussion
Application range
Nomenclature
 = pressure, psia
= pressure, psia = bubble point pressure, psia
= bubble point pressure, psia = oil gas ration, scf/stb
= oil gas ration, scf/stb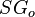 = oil specific gravity, dimensionless
= oil specific gravity, dimensionless = temperature, °F
= temperature, °F
 = viscosity, cP
= viscosity, cP
References
- ↑ Beggs, H. D.; Robinson, J. R. (September 1975). "Estimating the Viscosity of Crude Oil Systems"
 . Journal of Petroleum Technology. 27(09) (SPE-5434-PA).
. Journal of Petroleum Technology. 27(09) (SPE-5434-PA).
- ↑
Vazquez, M.; Beggs, H.D. (1980). "Correlations for Fluid Physical Property Prediction."
 . Society of Petroleum Engineers (SPE-6719-PA).
. Society of Petroleum Engineers (SPE-6719-PA).