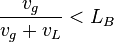Difference between revisions of "Griffith correlation"
From wiki.pengtools.com
(→References) |
|||
| (16 intermediate revisions by the same user not shown) | |||
| Line 8: | Line 8: | ||
The bubble flow exist when: | The bubble flow exist when: | ||
| − | :<math> \frac{ | + | :<math> \frac{v_g}{v_g + v_L} < L_B </math><ref name= Economides /> |
:<math> L_B = 1.071 - 0.2218 \frac{(v_g+v_L)^2}{D}</math>, with the limit <math> L_B \geqslant 0.13 </math><ref name= Orkiszewski /> | :<math> L_B = 1.071 - 0.2218 \frac{(v_g+v_L)^2}{D}</math>, with the limit <math> L_B \geqslant 0.13 </math><ref name= Orkiszewski /> | ||
| Line 16: | Line 16: | ||
== Discussion == | == Discussion == | ||
| + | |||
| + | [[Griffith correlation]] adds a hook to the originally straight [[Hagedorn and Brown]] [[VLP]] curve. | ||
| + | |||
== Nomenclature == | == Nomenclature == | ||
| + | |||
| + | :<math> D </math> = pipe diameter, ft | ||
| + | :<math> H_g </math> = gas holdup factor, dimensionless | ||
| + | :<math> L_B </math> = bubble-slug boundary, dimensionless | ||
| + | :<math> v_g </math> = gas velocity, ft/sec | ||
| + | :<math> v_L </math> = liquid velocity, ft/sec | ||
| + | :<math> v_s </math> = 0.8, slip velocity (difference between average gas and liquid velocities), ft/sec | ||
| + | |||
== References == | == References == | ||
<references> | <references> | ||
| Line 29: | Line 40: | ||
|volume=83 | |volume=83 | ||
|pages=307-320 | |pages=307-320 | ||
| + | |url=http://heattransfer.asmedigitalcollection.asme.org/article.aspx?articleid=1432339&resultClick=3 | ||
| + | |url-access= subscription | ||
}}</ref> | }}</ref> | ||
| Line 41: | Line 54: | ||
|url=https://www.onepetro.org/journal-paper/SPE-1546-PA | |url=https://www.onepetro.org/journal-paper/SPE-1546-PA | ||
|url-access= subscription | |url-access= subscription | ||
| + | }}</ref> | ||
| + | |||
| + | <ref name=Economides>{{cite book | ||
| + | |last1= Economides |first1=M.J. | ||
| + | |last2= Hill |first2=A.D. | ||
| + | |last3= Economides |first3=C.E. | ||
| + | |last4= Zhu |first4=D. | ||
| + | |title=Petroleum Production Systems | ||
| + | |edition=2 | ||
| + | |date=2013 | ||
| + | |publisher=Prentice Hall | ||
| + | |place=Westford, Massachusetts | ||
| + | |isbn=978-0-13-703158-0 | ||
}}</ref> | }}</ref> | ||
</references> | </references> | ||
[[Category:pengtools]] | [[Category:pengtools]] | ||
| − | [[Category: | + | [[Category:PQplot]] |
| + | |||
| + | {{#seo: | ||
| + | |title=Griffith correlation | ||
| + | |titlemode= replace | ||
| + | |keywords=Griffith correlation | ||
| + | |description=Griffith correlation is an empirical correlation which defines: the boundary between the bubble and slug flow. | ||
| + | }} | ||
Latest revision as of 09:27, 6 December 2018
Brief
The Griffith correlation [1] is an empirical correlation which defines:
- The boundary between the bubble and slug flow[2]
- The void fraction of gas in bubble flow - gas hold up Hg[2]
Math & Physics
The bubble flow exist when:
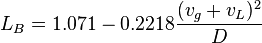, with the limit 
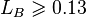[2]
The gas holdup:
Discussion
Griffith correlation adds a hook to the originally straight Hagedorn and Brown VLP curve.
Nomenclature
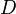= pipe diameter, ft
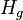= gas holdup factor, dimensionless
= bubble-slug boundary, dimensionless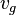
= gas velocity, ft/sec
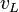= liquid velocity, ft/sec
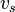= 0.8, slip velocity (difference between average gas and liquid velocities), ft/sec
References
- ↑ Griffith, P.; Wallis, G. B. (August 1961). "Two-Phase Slug Flow"
. Journal of Heat Transfer. ASME. 83: 307–320.
- ↑ 2.0 2.1 2.2 2.3 Orkiszewski, J. (June 1967). "Predicting Two-Phase Pressure Drops in Vertical Pipe". Journal of Petroleum Technology. SPE. 19 (SPE-1546-PA).
- ↑ Economides, M.J.; Hill, A.D.; Economides, C.E.; Zhu, D. (2013). Petroleum Production Systems (2 ed.). Westford, Massachusetts: Prentice Hall. ISBN 978-0-13-703158-0.
